溫馨提示×
您好,登錄后才能下訂單哦!
點擊 登錄注冊 即表示同意《億速云用戶服務條款》
您好,登錄后才能下訂單哦!
定義
并查集是一種樹型的數據結構,用于處理一些不相交集合(Disjoint Sets)的合并及查詢問題。常常在使用中以森林來表示。
應用
若某個朋友圈過于龐大,要判斷兩個人是否是在一個朋友圈,確實還很不容易,給出某個朋友關系圖,求任意給出的兩個人是否在一個朋友圈。 規定:x和y是朋友,y和z是朋友,那么x和z在一個朋友圈。如果x,y是朋友,那么x的朋友都與y的在一個朋友圈,y的朋友也都與x在一個朋友圈。
如下圖:
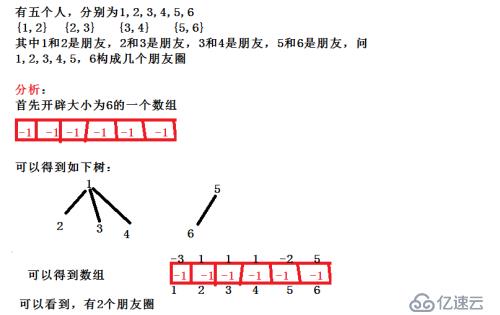
代碼:
//找朋友圈個數
//找父親節點
int FindRoot(int child1, int *_set)
{
int root = child1;
while (_set[root] >= 0)
{
root = _set[root];
}
return root;
}
//合并
void Union(int root1, int root2, int *&_set)
{
_set[root1] += _set[root2];
_set[root2] = root1;
}
int Friend(int n, int m, int r[][2])//n為人數,m為組數,r為關系
{
assert(n > 0);
assert(m > 0);
assert(r);
int *_set = new int[n];
for (int i = 0; i < n+1; i++)
{
_set[i] = -1;
}
for (int i = 0; i < m; i++)
{
int root1 = FindRoot(r[i][0],_set);
int root2 = FindRoot(r[i][1],_set);
if ((_set[root1] == -1 && _set[root2] == -1) || root1 != root2)
{
Union(root1, root2, _set);
}
}
int count = 0;
for (int i = 1; i <= n; i++)
{
if (_set[i] < 0)
{
count++;
}
}
return count;
}
//主函數
#define _CRT_SECURE_NO_WARNINGS 1
#include<iostream>
using namespace std;
#include<assert.h>
#include"UnionFindSet.h"
int main()
{
int r[][2] = { { 1, 2 }, { 2, 3 }, { 3, 4 }, { 5, 6 } };
cout << Friend(6, 4, r) << endl;
system("pause");
return 0;
}
免責聲明:本站發布的內容(圖片、視頻和文字)以原創、轉載和分享為主,文章觀點不代表本網站立場,如果涉及侵權請聯系站長郵箱:is@yisu.com進行舉報,并提供相關證據,一經查實,將立刻刪除涉嫌侵權內容。